Acoustics and Tunings

The process of making musical tones consists of producing regular vibrations in the air at an audible level. These vibrations may be caused by a string and a resonator, by a column of air, or by any object that vibrates at a given frequency. The fundamental waveform of a vibrating system, its slowest possible rate of vibration, is governed by the length and dimensions of the system. Dividing the length of a vibrating string in half produces a pitch one octave higher than the full length of the string. One-third of the length produces a pitch an octave plus a perfect fifth higher, and one-fourth of the original length produces a pitch two octaves higher. The first four vibrational nodes of a string fastened at both ends are shown in the diagram below.

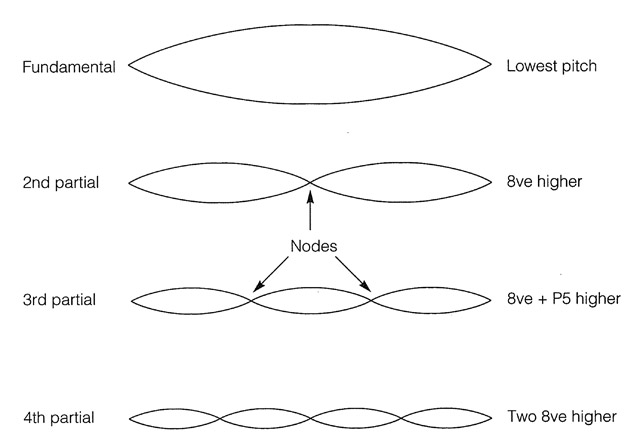
FIGURE 1.1 Fundamental and first three overtones of a vibrating string

Waves of pressure in a vibrating column of air act in much the same fashion as the vibrating string.  The nodes are points of greater pressure within the column. 

The composite waveform of any system is a combination of the fundamental and its multiples, or partials. In 1862 Hermann von Helmholtz demonstrated that the relative strength of the partials determines the tone color, or timbre, of a sound. It has since been proven that attack, decay, and release of a sound over time are prime factors in recognizing a sound source. Formant regions, or frequencies where certain harmonics are more pronounced, are also important factors in identifying sounds.

A pipe that is open at both ends acts differently than one closed at one or stopped. Both are similarly affected by holes drilled in the sides, which relieve the pressure at a length that determines the pitch of a tone. The resonance curve for strings and open pipes is shown below, the first nine partials present in decreasing strength.

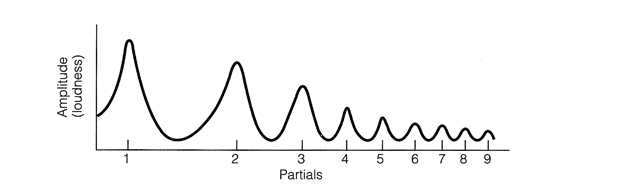
FIGURE 1.2 Resonance curve of strings and open pipes

The resonance curve for a pipe dosed at one end is shown below, with the odd partials much stronger.

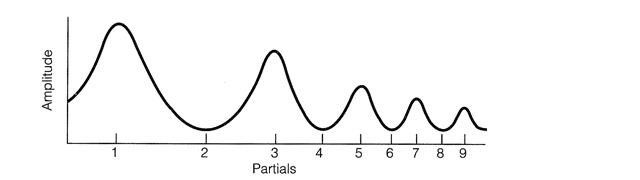
FIGURE 1.3 Resonance curve of a stopped pipe

Each of the partials of a vibrating system can be identified as a pitch in the overtone series. Knowledge of this series is relevant to understanding how most instruments function, particularly brass and strings. It is easy to remember the pitches in this harmonic series if they are related to the fundamental pitch as scale degrees in a major key. The second partial is an octave above the first partial, which is the fundamental, or tonic.  The third partial is a fifth in the major scale, the fourth partial another tonic, and the fifth partial is a third in the major scale. To find the scale degree from the ninth to the sixteenth partial, subtract seven from the number of the partial. For example, the thirteenth partial is the sixth scale degree.

FIGURE 1.4 Overtone series

In the sixth century B. C., the Greek scholar Pythagoras observed that if a string were divided in half, the sound of half the string was an octave above the original fundamental pitch. He converted this information into a mathematical ratio between the frequency of the partial compared to the fundamental and went on to provide a mathematical ratio for each interval, as shown below.

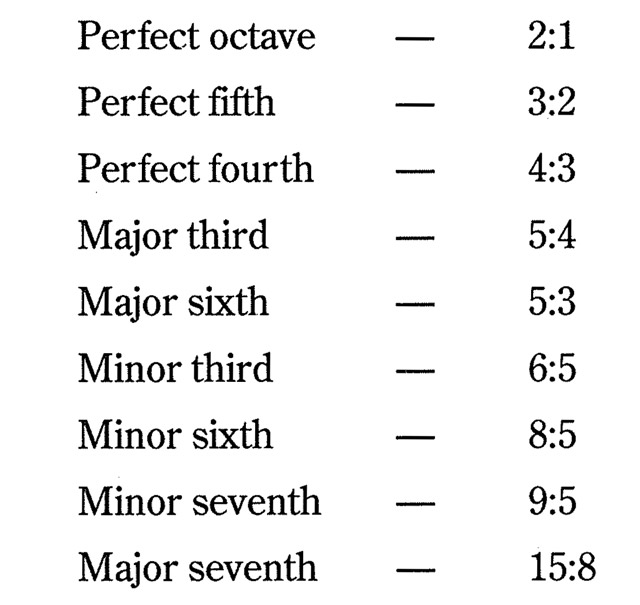
Pythagorean tuning refers to the use of the 3:2 ratio for perfect fifths to determine the frequency of pitches in the scale. Because tuning in this method is not consistent with the pitches found in the overtone series, it is not used currently. Just tuning, which amounts to tuning perfect fourths and fifths and then the thirds above them, provides only three major triads in tune with the overtone series. These are the tonic, sub dominant, and dominant chords of the key in which the instrument is tuned. Mean-tone tuning, a form of tempered just tuning, puts the thirds in tune and the fifths out of tune with the series. Equal temperament consists of twelve equal divisions, or semitones, to the octave. It is practical for keyboard instruments, but compared to the harmonic series, the seventh, eleventh, thirteenth, and fourteenth partials are quite out of tune. Equal temperament has become the accepted compromise for modulatory music involving keyboard and mallet instruments.